Fixing Early Life Failures Can Make Your MTBF Worse

 

Let’s say we 6 months of life data on 100 units. We’re charged with looking at the data and determine the impact of fixing the problems that caused the earliest failures.

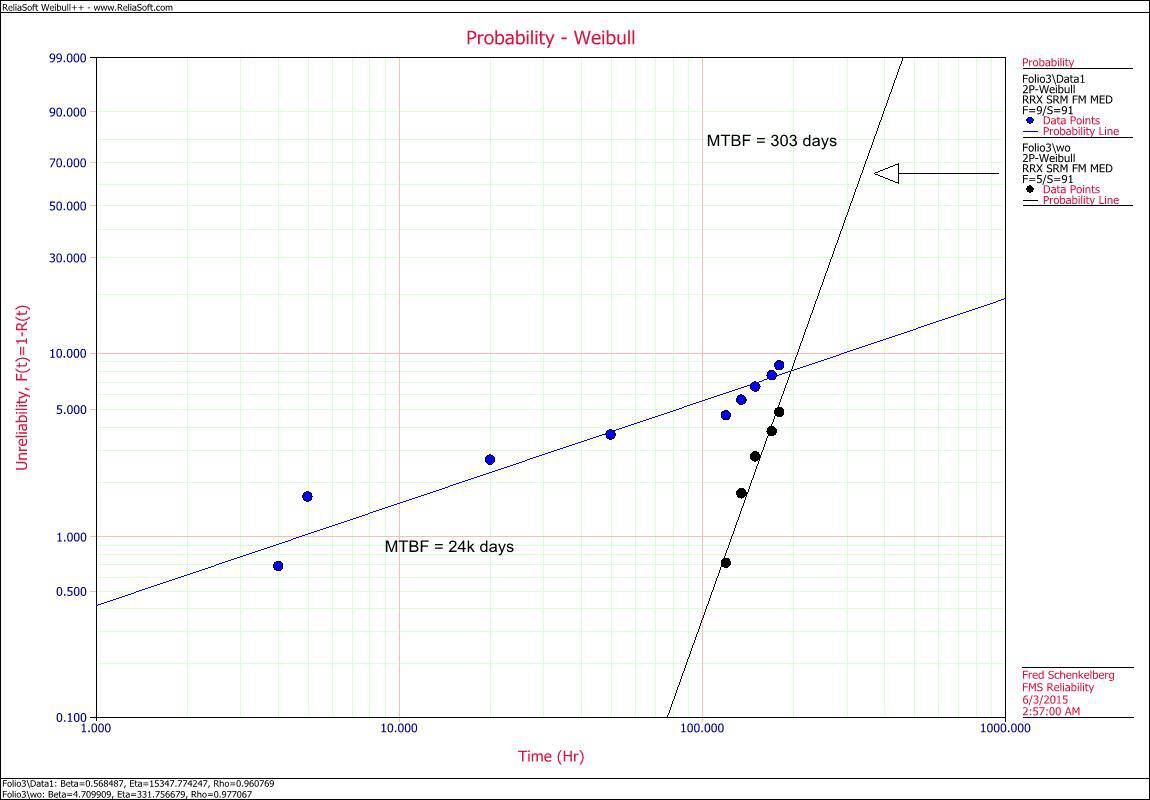
The initial look of the data includes 9 failures and 91 suspensions. Other then the nine all units operated for 180 days. The MTBF is about 24k days. Having heard about Weibull plotting and using the beta value as a guide initially find the blue line in the plot. The beta value is less than one so we start looking for supply chain, manufacturing or installation caused failures, as we suspect early life failures dominant the time to failure pattern.

Initial Steps to Improve the Product

Given clues and evidence that some of the products failed early we investigate and find evidence of damage to units during installation. In fact it appears the first four failures were due to installation damage. The fix will cost some money, so the director of engineer asks for an estimate of the effect of the change on the reliability of the system.

The organization uses MTBF as does the customer. The existing MTBF of 24k days exceeds the customers requirement of 10K days, yet avoiding early problems may be worth the customer good will. The motivation is driven by continuous improvement and not out of necessity or customer complaints.

Calculation of Impact of Change on Reliability

One way to estimate the effect of a removal of a failure mechanism is to examine the data without counting the removed failure mechanism. So, if the change to the installation practice in the best case completely prevents the initial four failures observed we are left with just the 5 other failures that occurred over the 6 months.

Removing the four initial failures and calculating MTBF we estimate MTBF will change to about 300 days.

Hum?

We removed failures and the MTBF got worse?

What Could Cause this Kind of Change?

The classic calculation for MTBF is the total time divided by the number of failures. Taking a closer look at time to failure behavior of the two different failure mechanisms may reveal what is happening. The early failures have a decreasing failure rate (Weibull beta parameter less than 1) over the first two months of operation. Later, in the last couple of months of operation, 5 failures occur and they appear to have an increasing rate of failure (Weibull beta parameter greater than 1).

By removing the four early failures the Weibull distribution fit changes from the blue line to the black line (steeper slope).

Recall that the MTBF value represents the point in time when about 63% of units have failed. With only 9 total failures out of 100 units we have only about 10% of units failed so the MTBF calculation is a projects to the future when most of have failed, it does not providing information about failures at 6 months or less directly.

In this case when the four early failures are removed the slope changed from about 0.7 to about 5, it rotated counter clockwise on the CDF plot.

If only using MTBF the results of removing four failures from the data made the measured MTBF much worse and would have prevented us from improving the product. By fitting the data to a Weibull distribution we learned to investigate early life failures, plus once that failure mechanism was removed revealed a potentially serious wear out failure mechanism.

This is an artificial example, of course, yet it illustrates the degree which an organization is blind to what is actually occurring by using only MTBF. Treat the data well and use multiple methods to understand the time to failure pattern.

The Reliability Metric

A Quick and Valuable Improvement Over MTBF

Finished it. 130 pages long and packed with advice on why and how to switch from MTBF to reliability.

Based in large part on comments, feedback, discussions and input from you, my peers in the NoMTBF tribe. Thanks for the encouragement and support.

The Reliability Metric book is available here. [Read more…]

Determine MTBF Given a Weibull Distribution

First off, not sure why anyone would want to do this, yet one of the issues I’ve heard concerning abandoning the use of MTBF is client ask for MTBF. If they will not accept reliability probabilities at specific durations, and insist on using MTBF, you probably should provide a value to them.

Let’s say you have a Weibull distribution model that described the time to failure distribution of your product. You’ve done the testing, modeling, and many field data analysis and know for the requestor’s application this is the best estimate of reliability performance. You can, quite easily calculate the MTBF value.

As you know, if theβ parameter is equal to one then the characteristic life, η, is equal to MTBF. If β is less than or greater than one, then use the following formula to determine the mean value, MTBF, for the distribution.

$latex \displaystyle&s=4 \mu =\eta \Gamma \left( 1+\frac{1}{\beta } \right)$

You’ll need the Gamma function and the Weibull parameters. The further β is from one, the bigger the difference between η and MTBF.

You can find a little more information and background at the article Calculate the Mean and Variance on the accendoreliability.com site under the CRE Preparation article series.

Spotted a Current Reference to Mil Hdbk 217 Recently

After a short convulsion of disbelieve I became shocked. This was  a guide for a government agency advising design teams and supporting reliability professional. It suggested using 217 to create the estimate of field reliability performance during the development phase.

Have we made not progress in 30 years?

What would do?

Let’s say you are reviewing a purchase contract and find a request for a reliability estimate based on Mil Hdbk 217F (the latest revision that is also been obsolete for many years), what would you do? Would you contact the author and request an update to the document? Would you pull to 217 and create the estimate? Would you work to create and estimate the reliability of a product using the best available current methods? Then convert that work to an MTBF and adjust the 217 inputs to create a similar result. Or would you ignore the 217 requirement and provide a reliability case instead?

Requirements are the requirements

When a customer demands a parts count prediction as a condition of the purchase, is that useful for either the development team or the customer?

No.

So, given the contract is signed and we are in the execution phase, what are your options?

1. Do the prediction and send over the report while moving on with other work.

2. Ask the customer to adjust the agreement to include a meaningful estimate.

3. Ignore the 217 requirement and provide a complete reliability case detailing the reliability performance of the product.

4. Find a new position that will not include MTBF parts count prediction.

The choice is yours.

I hope you would call out the misstep in the contract and help all parties get the information concerning reliability that they can actually use to make meaningful decisions.

MTBF in the Age of Physics of Failure

MTBF is the inverse of a failure rate, it is not reliability. Physics of failure (PoF) is a fundamental understanding and modeling of failure mechanisms. It’s the chemistry or physical activity that leads a functional product to fail. PoF is also not reliability.

Both MTBF and PoF have the capability to estimate or describe the time to failure behavior for a product. MTBF requires the knowledge of the underlying distribution of the data. PoF requires the use stresses and duration to allow a calculation of the expected probability of success over time.

MTBF start with a point estimate. PoF starts with the relationship of stress on the deterioration or damage to the material. One starts with time to failure data and consolidates into a single value, the other starts with determining the failure mechanism model.

Does MTBF has a Role Anymore?

Given the ability to model at the failure mechanism level even for a complex system, is there a need to summarize the time to failure information into a single value?

No.

MTBF was convenient when we had limited computing power and little understanding of failure mechanisms. Today, we can use the time to failure distributions directly. We can accommodate different stresses, different use pattern and thousands of potential failure mechanisms on a laptop computer.

MTBF has no purpose anymore. MTBF describes something we have and should have little interest in knowing.

Sure, PoF modeling takes time and resources to create. Sure, we may need complex mathematical models to adequately describe a failure mechanism. And, we may need to use simulation tools to estimate time to failure across a range of use and environmental conditions. Yet, it provide an estimate of reliability that is not possible using MTBF at any point in the process. PoF provides a means to support design and production decisions, to accommodate the changing nature of failure rates given specific experiences.

When will PoF become dominant?

When will we stop using MTBF? I think the answer to both is about the same time. It is going to happen when we, reliability minded professionals, decide to use the best available methods to create information that support the many decisions we have to make. PoF will become dominant soon. It provides superior information and superior decision, thus superior products. The market will eventually decide, and everyone will have to follow. Or, we can decide now to provide our customers reliable products.

We can help PoF become dominant by not waiting for it to become dominant.

Supply chain MTBF vs Reliability requirements

Let’s say you have a reliability goal for your product of 95% survive 2 years in an outdoor portable environment with the primary function of providing two way communication. There is an engineering reference specification detailing the product functions and requirements for performance. There is a complete document of environmental and use conditions . And you have similar detailed goals for the 1^st month of use the expected useful life of 5 years. [Read more…]

How to Estimate MTBF

Every now and then I receive an interesting question from a connection, colleague or friend. The questions that make me think or they discussion may be of value to you, I write a blog post.

In this case, there are a couple of interesting points to consider. Hopefully you are not facing a similar question. [Read more…]

What do we know with MTBF

How many times have you been given only MTBF, a single value? The data sheet or sales representative or website provides only MTBF and nothing more. We see it all the time, right? It is provided as the total answer to “what is the reliability performance expectation?”

So, given MTBF what do we really know about reliability?

As you may suspect, not much. [Read more…]

MTBF by Another Name…

MTxx or MTxxx. I’ve lost count of how many variations of MTBF there exist.

MTBUR is mean time between unscheduled replacements, for example.

I’m sure you have a short list in mind already of your organizations or industries set of metrics. [Read more…]

Time to move on from Mean Time Between Failure (MTBF) and Mean Time To Failure (MTTF)

Guest Post by Dan Burrows

Reliability, Quality, Six Sigma, & Performance Improvement Leader

The reliability profession has historically embraced two metrics, Mean Time Between Failure (MTBF) for repairable items and Mean Time To Failure (MTTF) for non-repairable items. They did this mostly out of convenience when dealing with large populations such as fleets of vehicles to address the random failures that were being experienced and to make the mathematics simple. And this approach worked fairly well before better approaches came into play. But this approach also worked fairly well because other reliability and maintainability practices were also enforced, namely planned/preventive/scheduled maintenance whereby serviceable items were serviced to keep them in proper operating condition, wearable items were replaced or restored, life limited items were replaced and good operating and failure data was kept. Without enforcing the maintainability and good data side of this, MTBF and MTTF become misleading at the least and dangerous in many cases.

Thus, MTBF or MTTF could address the flat portion of the traditional “Bathtub Curve”. Proper maintenance could address the wearout/life limit portion of the bathtub curve. And screening and run in/burn in could mitigate the early failure portion of the bathtub curve.

Traditional Bathtub Curve

So, there are four big mistakes that people often make with MTBF and MTTF related to the bathtub curve:

Mistake #1: MTBF and MTTF are erroneously used as projections of product useful life.

Mistake #2: MTBF and MTTF assume a constant failure rate during the useful life of the item.

Mistake #3: MTBF and MTTF are given an assumption of high likelihood that the product will make it to the value.

Mistake #4: MTBF and MTTF data is assumed to be good and current.

Let’s take a closer look at these four big mistakes…

Mistake #1: MTBF and MTTF are erroneously used as projections of product useful life

Let’s take a common example. Electrolytic capacitors can have MTBF (actually should be stated MTTF since they are not repairable) values of 10⁸ (one hundred million) or 10⁹ (one billion) hours. If one were to divide these numbers by hours in a year to project useful life, this would result in a useful life of 11,415 to 114,155 years! In reality, electrolytic capacitors, if derated and applied properly typically have a useful life of 10 to 20 years. This is because the electrolyte in electrolytic capacitors dissipates, drying up the capacitor, causing significant degradation in performance (capacitance, leakage current, or ESR) or outright open or short failure. This doesn’t mean that electrolytic capacitors are necessarily bad, just that they don’t live for 10,000+ years.

So, how should MTBF and MTTF be used? They should be used as indicators of failure rate during the useful life of the product. So, you take the MTBF or MTTF value and invert it, dividing 1 by it. This gives you the expected failure rate per operating hour for the product during its useful life. So, our electrolytic capacitors that have a MTBF of 10⁸ (one hundred million) or 10⁹ (one billion) hours actually have an expected failure rate of 1 to 10 x 10^-9 failures per operating hour. It is possible that they will be very reliable during their 10 to 20 year useful life, but then they are dried out and done.

Using MTBF or MTTF values as projections of product useful life is extremely misleading and will probably get you laughed out of your job. Think about that before you improperly use MTBF or MTTF to claim that a product will last 10,000 years. Somebody may ask for a warranty that long. In writing.

Mistake #2: MTBF and MTTF assume a constant failure rate during the useful life of the item.

Many products do not exhibit a constant failure rate. Especially if the early failures were not mitigated and the product was not properly maintained. MTBF and MTTF only address the portion of the product’s failure population that arise out of random chance and apply a very simplistic “mean” by dividing the total operating time of the product population by the total number of failures. This is then made to look scientific by then stating that this is an exponential distribution whereby the failures that arose in the population were evenly distributed with no proof of even distribution. But the world is not random and failures do not arrive at a constant rate over the life of the product or product population. Most product failures happen in non-exponential distribution, non-random patterns for identifiable reasons.

Let’s say you have a product population of five products with the following failure times: 98, 99, 100, 101, 102. If you use the standard MTBF averaging, you have a MTBF of 100 hours. But these failures are not randomly distributed with a constant failure rate. They are clustered around 100 hours and there is probably an identifiable reason why.

Let’s say you have a product population of five products with the following failure times: 10, 10, 10, 235, 235. Again, if you use the standard MTBF averaging, you have a MTBF of 100 hours. It is obvious that there is something going on that caused three products to have a very short life and two products to have a much longer life. Either way, there is probably an identifiable reason why three products failed early and two lived much longer.

Assuming a constant failure rate and using simple averaging of failure times to come up with MTBF or MTTF values is lazy at best. Don’t be lazy, investigate failures to find root causes. These root causes will help you determine how to design products to eliminate the failure, mitigate against the failure, or perform proper preventive and predictive maintenance to avoid the failure.

Mistake #3: MTBF and MTTF are given an assumption of high likelihood that the product will make it to the value.

Even if we do mitigate early life failures and perform proper maintenance, most people assume that the MTBF or MTTF is a value with high statistical likelihood like a B10 life (the point at which 10% of products fail and 90% continue to survive) for bearings. Due to the constant failure rate assumption and underlying statistical distribution, MTBF and MTTF are actually the point at which 63% of products would have failed and only 37% survive. Some high likelihood, — recall that MTBF is the inverse of the failure rate, not a duration.

You can check the math yourself. The probability of survival of a product following the constant failure rate of the exponential distribution is e^{-(1/MTBF)(Operating Time).} So, a product with a MTBF of 200,000 hours will have a probability of survival of e^{-(1/200,000)(200,000)} or 37%.

Assuming MTBF and MTTF are high likelihood projections is actually almost the exact opposite of how the math really works out. Use MTBF and MTTF with high caution, not high trust.

Mistake #4: MTBF and MTTF data is assumed to be good and current

Even if you make it past the first three mistakes, this fourth mistake usually throws a wrench in MTBF and MTTF because many of the prediction models and prediction tools being sold are based on outdated information and outdated technologies. One example of this is using a MTBF prediction model for a flash memory device. Most of the data behind prediction tools stopped getting updated when the United States Defense Department transitioned to commercial off the shelf acquisition practices and stopped funding the collection of component operating and failure data. One example is many models for flash memory include devices that have 256K or 512K capacity while the world has moved way past this.

Assuming that the information in prediction models and tools is good and current may lead you to making extremely erroneous predictions of MTBF and MTTF. If you are going to predict MTBF or MTTF, you need to either have collected the operating and failure data yourself and analyzed it properly or make sure that component suppliers are providing good data.

Time to move on…

MTBF and MTTF may have had a brief time in the spotlight of reliability when items were screened for early defects and maintained properly, good data was kept, and people didn’t know how to or didn’t know better about uncovering root causes of failures and designing them out or mitigating them. But that past is past. It is time to move on from MTBF and MTTF to more effective methods to drive reliability.

Maybe you are one of the lucky ones who deal with large product populations, products are all properly maintained, and you keep good data so the MTBF and MTTF math still holds.

Good for you.

Most of us live in a demanding world with demanding customers and demanding bosses and tight schedules and limited resources. Customers don’t want to hear about averages that have low confidence levels, they expect the product they bought to live its expected usage life. Bosses don’t want to hear about the huge number of product samples needed to test and huge amount of field data needed to statistically derive the proper failure distribution analysis, they want to know why the product has not launched yet.

Reliability professionals in today’s world have to understand more and guide product teams to:

Design for Reliability for proper application, design margin, and derating.

Design for Maintainability to address issues that must be mitigated by maintenance when the needed product life reliability cannot be achieved without maintenance actions.

Failure Mode and Effects Analysis (FMEA) and Fault Tree Analysis (FTA) to determine the risks to the product based on severity, occurrence, and detection to drive actions to drive down risk before it becomes realized.

Reliability Testing to aggressively test and discover failures, at what point failures occur, and how much reliability margin the product will have to drive actions to correct the weak links in the design.

Design for Manufacturability to preserve the designed in reliability of the product during its manufacture.

Get Good Data from your own test and field history and supplier data you can trust instead of relying on generic and often outdated and obsolete prediction data. Data for your products in your customer’s hands tells you the real story of how your products are actually performing in their actual (and sometimes surprising) usage applications and operating environments.

 

Everybody Uses MTBF, Really?

I don’t.

When you say ‘… uses MTBF’? What is it you’re implying? Do they make important business decisions, or assess product designs, or order spares based on using MTBF?

Probably not.

When you use MTBF, what do you use it to accomplish?

• Do you write a report and send it to the requesting team?
• Do you run a calculation and provide the resulting MTBF to customers or vendors?
• Do you or anyone in your organization use MTBF in a useful manner.

And, if so, does it work for you? Does MTBF actually provide a useful metric related to your product’s reliability performance?

In my experience, MTBF and related metrics are great for meeting requirements or fulfilling requests, not much else. They are not useful for decision making. MTBF is next to useless when ordering spares. And, it is so commonly misunderstood that the report values are often simply misleading.

Do you receive request for MTBF from customers or internal teams? What do they use MTBF to accomplish? Check of as done?

Some may claim they use MTBF as a comparison to previous products. Some claim it provides an insights to the expected reliability performance. Some really do not know what do with MTBF so just ignore the value.

When gathering data for a part count prediction (aka Mil Hdbk 217 or similar) do you request MTBF values? Is so, do you also ask about failure mechanisms, derating parameters, or how/what will most likely fail?

Simply taking the MTBF value provided reinforces that notion that ‘everybody uses MTBF’ and does not provide you or your team useful information.

Data sheets, vendor websites, reliability reports, etc. all contain MTBF (sometimes called life, or reliability, yet the most common reported metric is MTBF or something similar).

MTBF is around us, built into tools, and expected. My contention is that even though it is not useful, it is so common, that it is assumed everybody uses MTBF.

Don’t be Everybody.

You will do a better job reporting reliability as couplets of probability of success and duration, rather than MTBF.

Do something that is useful and easy to use. Do not use MTBF. Be better than everybody. Add value to your organization, to your team, to your customers. Help others by your example, to be like you and not like everybody else.

I’m on vacation and this is just a quick post for the week. The top five posts of NoMTBF.com by visits to date.

How to Calculate MTTF is probably popular as folks may be searching for a way to do this calculation. It’s actually very simple, yet this article asks why would you want to calculate MTBF?

Set a Reliability Goal without MTBF is another recent article and may have gather interest given it may seem impassable to set a goal without MTBF. It is possible and actually useful.

Why The Drain the The Bathtub Curve Matters is a guest post by Kirk Grey. He explores one of the many myths around the common bathtub curve and modern products.

What is the purpose of Reliability Predictions is a guest post by Andrew Roland (3 of the top 5 are guest posts…) where he examines the useful use of predictions and where many have gone astray assuming a use.

Where does 0.7eV Come From – well, actually the activation energy that represents a doubling of rate in a chemical reaction with an increase in temperature of about 10°C is 0.7eV – beyond that Kirk explores the ramifications.

Back at my office next week, and home to find a few more record breaking articles to post. Plus, if you’re interesting in writing a post, either a problem with solution, a case study, or common issue with assumptions related to reliability – let’s see how it does with visits and views.

Ease and Joy of MTBF Calculation

Mean time between failure, MTBF is a common reliability metric. It is easy to calculate. It is regularly requested and readily offered.

MTBF also does not contain very much information of any use. [Read more…]

Resist the temptation to Use MTBF

Sure, it would be easy to use MTBF for a system reliability goal. Your organization has regularly used MTBF. Your customers are asking for MTBF. The competition all use MTBF. Even your vendors supply only MTBF.

Yet, you know it’s not the best metric to use. It’s not accurate, it’s not useful, and you rather use something else.

Yeah! [Read more…]