Introduction
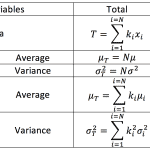
In some of my articles, I have referred to The Central Limit Theorem, a development in probability theory. It can be stated
“When independent identically distributed random variables are added, their normalized sum tends toward a normal distribution (informally a “bell curve”) even if the original variables themselves are not normally distributed.”
We can apply this principle to many practical problems to analyze the distribution of the sample mean. In this article, I provide graphical and mathematical descriptions and a practical example.